The Anatomy of the Eye
The human eye is a complex anatomical device that remarkably demonstrates the architectural wonders of the human body. Like a camera, the eye is able to refract light and produce a focused image that can stimulate neural responses and enable the ability to see. In Lesson 6, we will focus on the physics of sight. We will use our understanding of refraction and image formation to understand the means by which the human eye produces images of distant and nearby objects. Additionally, we will investigate some of the common vision problems that plague humans and the customary solutions to those problems. As we proceed through Lesson 6, we will apply our understanding of refraction and lenses to the physics of sight.
The eye is essentially an opaque eyeball filled with a water-like fluid. In the front of the eyeball is a transparent opening known as the cornea. The cornea is a thin membrane that has an index of refraction of approximately 1.38. The cornea has the dual purpose of protecting the eye and refracting light as it enters the eye. After light passes through the cornea, a portion of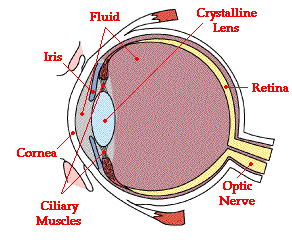 
it passes through an opening known as the pupil. Rather than being an actual part of the eye's anatomy, the pupil is merely an opening. The pupil is the black portion in the middle of the eyeball. Its black appearance is attributed to the fact that the light that the pupil allows to enter the eye is absorbed on the retina (and elsewhere) and does not exit the eye. Thus, as you sight at another person's pupil opening, no light is exiting their pupil and coming to your eye; subsequently, the pupil appears black.
it passes through an opening known as the pupil. Rather than being an actual part of the eye's anatomy, the pupil is merely an opening. The pupil is the black portion in the middle of the eyeball. Its black appearance is attributed to the fact that the light that the pupil allows to enter the eye is absorbed on the retina (and elsewhere) and does not exit the eye. Thus, as you sight at another person's pupil opening, no light is exiting their pupil and coming to your eye; subsequently, the pupil appears black.
Like the aperture of a camera, the size of the pupil opening can be adjusted by the dilation of the iris. The iris is the colored part of the eye - being blue for some people and brown for others (and so forth); it is a diaphragm that is capable of stretching and reducing the size of the opening. In bright-light situations, the iris adjusts its size to reduce the pupil opening and limit the amount of light that enters the eye. And in dim-light situations, the iris adjusts so as to maximize the size of the pupil opening and increase the amount of light that enters the eye.
Light that passes through the pupil opening, will enter the crystalline lens. The crystalline lens is made of layers of a fibrous material that has an index of refraction of roughly 1.40. Unlike the lens on a camera, the lens of the eye is able to change its shape and thus serves to fine-tune the vision process. The lens is attached to the ciliary muscles. These muscles relax and contract in order to change the shape of the lens. By carefully adjusting the lenses shape, the ciliary muscles assist the eye in the critical task of producing an image on the back of the eyeball.
The inner surface of the eye is known as the retina. The retina contains the rods and cones that serve the task of detecting the intensity and the frequency of the incoming light. An adult eye is typically equipped with up to 120 million rods that detect the intensity of light and about 6 million cones that detect the frequency of light. These rods and cones send nerve impulses to the brain. The nerve impulses travel through a network of nerve cells. There are as many as one million neural pathways from the rods and cones to the brain. This network of nerve cells is bundled together to form the optic nerve on the very back of the eyeball.
Each part of the eye plays a distinct part in enabling humans to see. The ultimate goal of such an anatomy is to allow humans to focus images on the back of the retina.
1/f = 1/do + 1/di
hi/ho = - di/do
Image Formation and Detection
Earlier in Lesson 6, we learned that the eye consists of a cornea (thin outer membrane), a lens attached to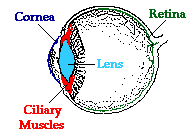 
ciliary muscles, and a retina (inner surface equipped with nerve cells). These four parts of the eye are the most instrumental in the task of producing images that are discernible by the brain. In order to facilitate the ability to see, each part must enable the eye to refract light so that is produces a focused image on the retina.
ciliary muscles, and a retina (inner surface equipped with nerve cells). These four parts of the eye are the most instrumental in the task of producing images that are discernible by the brain. In order to facilitate the ability to see, each part must enable the eye to refract light so that is produces a focused image on the retina.
It is a surprise to most people to find out that the lens of the eye is not where all the refraction of incoming light rays takes place. Most of the refraction occurs at the cornea. The cornea is the outer membrane of the eyeball that has an index of refraction of 1.38. The index of refraction of the cornea is significantly greater than the index of refraction of the surrounding air. This difference in optical density between the air the corneal material combined with the fact that the cornea has the shape of a converging lens is what explains the ability of the cornea to do most of the refracting of incoming light rays. The crystalline lens is able to alter its shape due to the action of the ciliary muscles. This serves to induce small alterations in the amount of corneal bulge as well as to fine-tune some of the additional refraction that occurs as light passes through the lens material.
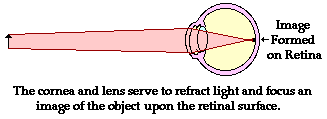
The bulging shape of the cornea causes it to refract light in a manner to similar to a double convex lens. The focal length of the cornea-lens system varies with the amount of contraction (or relaxation) of the ciliary muscles and the resulting shape of the lens. In general, the focal length is approximately 1.8 cm, give or take a millimeter. As learned in our discussion of convex lenses in Lesson 5, the image location, size, orientation, and type is dependent upon the location of the object relative to the focal point and the 2F point of a lens system. Since the object is typically located at a point in space more than 2-focal lengths from the "lens," the image will be located somewhere between the focal point of the "lens" and the 2F point. The image will be inverted, reduced in size, and real. Quite conveniently, the cornea-lens system produces an image of an object on the retinal surface. The process by which this occurs is known as accommodation and will be discussed in more detail in the next part of Lesson 6. Fortunately, the image is a real image - formed by the actual convergence of light rays at a point in space. Vision is dependent upon the stimulation of nerve impulses by an incoming light rays. Only real images would be capable of producing such a stimulation. Finally, the reduction in the size of the image allows the entire image to "fit" on the retina. The fact that the image is inverted poses no problem. Our brain has become quite accustomed to this and properly interprets the signal as originating from a right-side-up object.
The use of the lens equation and magnification equation can provide an idea of the quantitative relationship between the object distance, image distance and focal length. For now we will assume that the cornea-lens system has a focal length of 1.80 cm (0.0180 m). We will attempt to determine the image size and image location of a 6-foot tall man (ho=1.83 m) who is standing a distance of approximately 10 feet away (do= 3.05 meters). (The lens equation is derived geometrically upon the assumption that the lens is a thin lens. The lens of the eye is anything but thin and as such the lens equation does not provide a truly accurate model of the eye lens. Despite this fact, we will use the equation as a simplified approximation of the mathematics of the eye.)
Next identify the unknown quantities that you wish to solve for.
To determine the image distance, the lens equation can be used. The following lines represent the solution to the image distance; substitutions and algebraic steps are shown.
1/(0.0180 m) = 1/(3.05 m) + 1/di
55.6 m-1 = 0.328 m-1 + 1/di
55.2 m-1 = 1/di
To determine the image height, the magnification equation is needed. Since three of the four quantities in the equation (disregarding the M) are known, the fourth quantity can be calculated. The solution is shown below.
hi /(1.83 m) = - ( 0.0181 m)/(3.05 m)
hi = - (1.83 m) • (0.0181m)/(3.05 m)
From the calculations in this problem it can be concluded that if a 1.83-m tall person is standing 3.05 m from your cornea-lens system having a focal length of 1.8 cm, then the image will be inverted, 1.09-cm tall (the negative values for image height indicate that the image is an inverted image) and located 1.81 cm from the "lens".
Now of course, if the person is standing further away (or closer to) the eye, the image size and image distance will be adjusted accordingly. This is illustrated in the following table for the same 6-foot tall (1.83 m) person.
|
The results of these calculations (as illustrated in the table above) illustrate two important principles concerning the ability of the eye to discern objects that are both close up and far away. First, the distance between the observer and the object will greatly influence the image size (height and width of the image formed on the retina) and quality. Objects that are viewed at close proximity produce images that are larger than distant objects. Such an image is typically spread over the entirety of the retina and even at times would extend beyond the extremities of the retina. Thus, the full dimensions of a 6-foot tall person cannot be seen if he/she stands 1-meter away (unless the eyeballs are rolled in their socket). On the other hand, the entire image of the same person can easily be seen when standing 100-meters away. In this instance, the image takes up a small amount of space on the back of the retina. The drawback however is that the finer details of the image are lost due to the reduction in the size of the image. Since the image is stimulating a smaller region of nerve cells, some details are lost since they fail to provide sufficient stimulation to allow the brain to discern them. For such an object distance, the small image that is created of a man might make it impossible to discern that the man's fly is open or that his socks and belt don't match.
Second, the varying distance between the observer and the object poses some potential problems for the human eye. Objects located varying distances from a lens system with a fixed focal length produce images that are varying distances from the lens. Yet, the eye must always produce an image on the retina - a location that is always the same distance away from the cornea. The eye cannot afford to allow changes in the image distance. So how does an eye always focus images with the same dimage regardless of the fact that the dobject is different? How can an object 100 meters away be focused the same distance from the cornea-lens system as an object that is 1 meter away? The answer: the cornea-lens system is able to change its focal length. The ciliary muscles of the eye serve to contract and relax, thus changing the shape of the lens
The Wonder of Accommodation
While the entire surface of the retina contains nerve cells, there is a small portion with a diameter of approximately 0.25 mm where the concentration of rods and cones is greatest. This region, known as thefovea centralis, is the optimal location for the formation of the image. The eye typically rotates in its socket in order to focus images of objects at this location. The distance from the outer surface of the cornea (where the light undergoes most of its refraction) to the central portion of the fovea on the retina is approximately 2.4 cm. Light entering the cornea must produce an image with a distance of 2.4 cm from its outer edge. Unlike a camera, which has the ability to change the distance between the film (the detector) and the lens, the distance between the retina (the detector) and the cornea (the refractor) is fixed. The image distance is unchangeable. Subsequently, the eye must be able to alter the focal length in order to focus images of both nearby and far away objects upon the retinal surface. As the object distance changes, the focal length must be changed in order to keep the image distance constant.
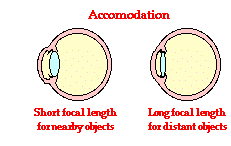The ability of the eye to adjust its focal length is known as accommodation. Since a nearby object (small dobject) is typically focused at a further distance (large dimage), the eye accommodates by assuming a lens shape that has a shorter focal length. This reduction in focal length will cause more refraction of light and serve to bring the image back closer to the cornea/lens system and upon the retinal surface. So for nearby objects, the ciliary muscles contract and squeeze the lens into a more convex shape. This increase in the curvature of the lens corresponds to a shorter focal length. On the other hand, a distant object (large dobject) is typically focused at a closer distance (small dimage). The eye accommodates by assuming a lens shape that has a longer focal length. So for distant objects the ciliary muscles relax and the lens returns to a flatter shape. This decrease in the curvature of the lens corresponds to a longer focal length.The data table below demonstrates how a changing focal length would be required to maintain a constant image distance of 1.80 cm.
|
(The values above have been calculated using the lens equation. The lens equation represents a simplified mathematical model of the eye.)
The ability of the eye to accommodate is automatic. Furthermore, it occurs instantaneously. Focus on a far away object and quickly turn your attention to a nearby object; observe that there is no noticeable delay in the ability of the eye to bring the nearby object into focus. Accommodation is a remarkable feat!
The power of a lens is measured by opticians in a unit known as a diopter. A diopter is the reciprocal of the focal length.
diopters = 1/(focal length)
A lens system with a focal length of 1.8 cm (0.018 m) is a 56-diopter lens. A lens system with a focal length of 1.68 cm is a 60-diopter lens. A healthy eye is able to bring both distant objects and nearby objects into focus without the need for corrective lenses. That is, the healthy eye is able to assume both a small and a large focal length; it would have the ability to view objects with a large variation in distance. The maximum variation in the power of the eye is called the Power of Accommodation. If an eye has the ability to assume a focal length of 1.80 cm (56 diopters) to view objects many miles away as well as the ability to assume a 1.68 cm focal length to view an object 0.25 meters away (60 diopters), then its Power of Accommodation would be measured as 4 diopters (60 diopters - 56 diopters).
The healthy eye of a young adult has a Power of Accommodation of approximately 4 diopters. As a person grows older, the Power of Accommodation typically decreases as a person becomes less able to view nearby objects. This failure to view nearby objects leads to the need for corrective lenses. In the next two sections of Lesson 6, we will discuss the two most common defects of the eye - nearsightedness and farsightedness.
Farsightedness and its Correction
The human eye's ability to accommodate allows it to view focused images of both nearby and distant objects. As mentioned earlier in Lesson 6, the lens of the eye assumes a large curvature (short focal length) to bring nearby objects into focus and a flatter shape (long focal length) to bring a distant object into focus. Unfortunately, the eye's inability to a provide a wide variance in focal length leads to a variety of vision defects. Most often, the defect occurs at one end of the spectrum - either the inability to assume a short focal length and focus on nearby objects or the inability to assume a long focal length and thus focus on distant objects.
mentioned earlier in Lesson 6, the lens of the eye assumes a large curvature (short focal length) to bring nearby objects into focus and a flatter shape (long focal length) to bring a distant object into focus. Unfortunately, the eye's inability to a provide a wide variance in focal length leads to a variety of vision defects. Most often, the defect occurs at one end of the spectrum - either the inability to assume a short focal length and focus on nearby objects or the inability to assume a long focal length and thus focus on distant objects.
Farsightedness or hyperopia is the inability of the eye to focus on nearby objects. The farsighted eye has no difficulty viewing distant objects. But the ability to view nearby objects requires a different lens shape - a shape that the farsighted eye is unable to assume. Subsequently, the farsighted eye is unable to focus on nearby objects. The problem most frequently arises during latter stages in life, as a result of the weakening of the ciliary muscles and/or the decreased flexibility of the lens. These two potential causes leads to the result that the lens of the eye can no longer assume the high curvature that is required to view nearby objects. The lens' power to refract light has diminished and the images of nearby objects are focused at a location behind the retina. On the retinal surface, where the light-detecting nerve cells are located, the image is not focused. These nerve cells thus detect a blurry image of nearby objects.
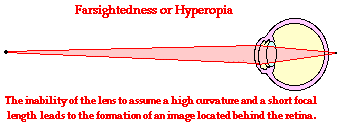
The cure for the farsighted eye centers around assisting the lens in refracting the light. Since the lens can no longer assume the convex and highly curved shape that is required to view nearby objects, it needs some help. Thus, the farsighted eye is assisted by the use of a converging lens. This converging lens will refract light before it enters the eye and subsequently decreases the image distance. By beginning the refraction process prior to light reaching the eye, the image of nearby objects is once again focused upon the retinal surface.
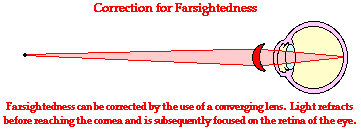
While farsightedness most often occurs among adults, occasionally younger people will suffer from this vision defect. When farsightedness occurs among youth, the cause is seldom related to the inability of the lens to assume a short focal length. In this case, the problem is more closely related to an eyeball that is shortened. Because the eyeball is shortened, the retina lies closer than usual to the cornea and lens. As a result, the image of nearby objects is formed beyond the retina. The traditional correction for such a problem is the same as for adults - the use of a converging lens.
Nearsightedness and its Correction
The human eye's ability to accommodate allows it to view focused images of both nearby and distant objects. As mentioned earlier in Lesson 6, the lens of the eye assumes a large curvature (short focal length) to bring nearby objects into focus and a flatter shape (long focal length) to bring a distant object into focus. Unfortunately, the eye's inability a wide variance in focal length leads to a variety of vision defects. Most often, the defect occurs at one end of the spectrum - either the inability to assume a short focal length and focus on nearby objects or the inability to assume a long focal length and thus focus on distant objects.
mentioned earlier in Lesson 6, the lens of the eye assumes a large curvature (short focal length) to bring nearby objects into focus and a flatter shape (long focal length) to bring a distant object into focus. Unfortunately, the eye's inability a wide variance in focal length leads to a variety of vision defects. Most often, the defect occurs at one end of the spectrum - either the inability to assume a short focal length and focus on nearby objects or the inability to assume a long focal length and thus focus on distant objects.
Nearsightedness or myopia is the inability of the eye to focus on distant objects. The nearsighted eye has no difficulty viewing nearby objects. But the ability to view distant objects requires that the light be refracted less. Nearsightedness will result if the light from distant objects is refracted more than is necessary. The problem is most common as a youth, and is usually the result of a bulging cornea or an elongated eyeball. If the cornea bulges more than its customary curvature, then it tends to refract light more than usual. This tends to cause the images of distant objects to form at locations in front of the retina. If the eyeball is elongated in the horizontal direction, then the retina is placed at a further distance from the cornea-lens system. Subsequently the images of distant objects form in front of the retina. On the retinal surface, where the light-detecting nerve cells are located, the image is not focused. These nerve cells thus detect a blurry image of distant objects.
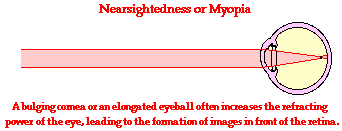
The cure for the nearsighted eye is to equip it with a diverging lens. Since the nature of the problem of nearsightedness is that the light is focused in front of the retina, a diverging lens will serve to diverge light before it reaches the eye. This light will then be converged by the cornea and lens to produce an image on the retina.
(Note: In the diagram above that the light approaching the eye from a distant object is traveling as a bundle of rays that are roughly parallel to each other. Suppose for a moment that the distant object is the lettering on the chalk board in the front of the room as you sight at it from the back of the room. Geometrically, whatever light rays from a particular letter or word that reach your eye will be traveling roughly parallel to each other. This is not the case when viewing nearby objects as demonstrated
0 التعليقات:
إرسال تعليق